3.1 Bedingte Wharscheinlichekeit und Unabhängigkeit
3.1. Bedingte Wharshceinlichkeit : 조건부확률, 알파벳작은/뒤에오는/분모의 사건이 우선순위.
Bedingte Wahrscheinlichkeit für \( \mathbf{A}\) falls \( \mathbf{B}\) bereits eingetreten ist: B가 우선순위. 먼저 일어난 사건
$$ {\mathbf{P}}_{B}(A) = \mathbf{P}(A|B) = \cfrac{\mathbf{P}(A \cap B)}{\mathbf{P}(B)} $$
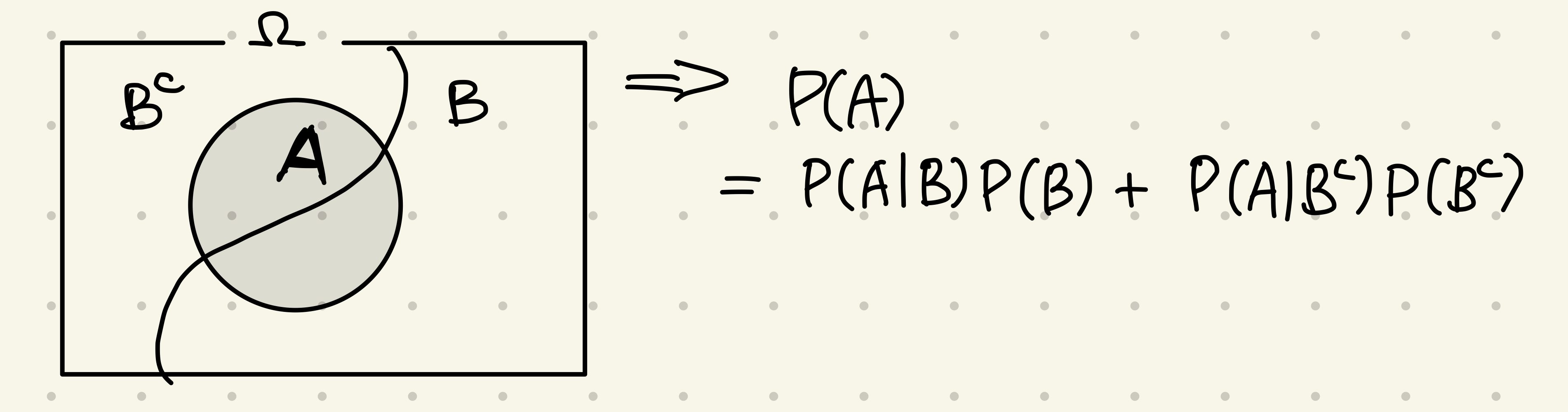
3.1.1 Totale Wahrscheinlichkeit und Satz von Bayes
Es muss gelten : Teilmengen \(\mathbf{B}_i \) sind disjunkt und decken ganz \( \Omega \) ab.
\( \rightarrow {\bigcup}_{i \in I}^{B_i} = \Omega \) für \( \mathbf{B}_i \cap \mathbf{B}_j = \emptyset , \forall i \neq j \)
Totale Wahrscheinlichekeit:
$$ \mathbf{P}(A) = \sum_{i \in I}\mathbf{P} (A|B_i) \mathbf{P}(B_i) $$
$$ \mathbf{P}(A) = \mathbf{P}(A|B_1)\mathbf{P}(B_1) + \mathbf{P}(A|B_2)\mathbf{P}(B_2) + ... + \mathbf{P}(A|B_k)\mathbf{P}(B_k) $$
Satz von Bayes:
$$ \mathbf{P}(B_k|A) = \cfrac{\mathbf{P}(A|B_k)\mathbf{P}(B_k)}{ \sum_{i \in I} \mathbf{P}(A|B_i)\mathbf{P}(B_i)} $$
$$ = \cfrac{\mathbf{P}(A|B_k)\mathbf{P}(B_k)}{\mathbf{P}(A)} $$
3.1.2 Multiplikationssatz
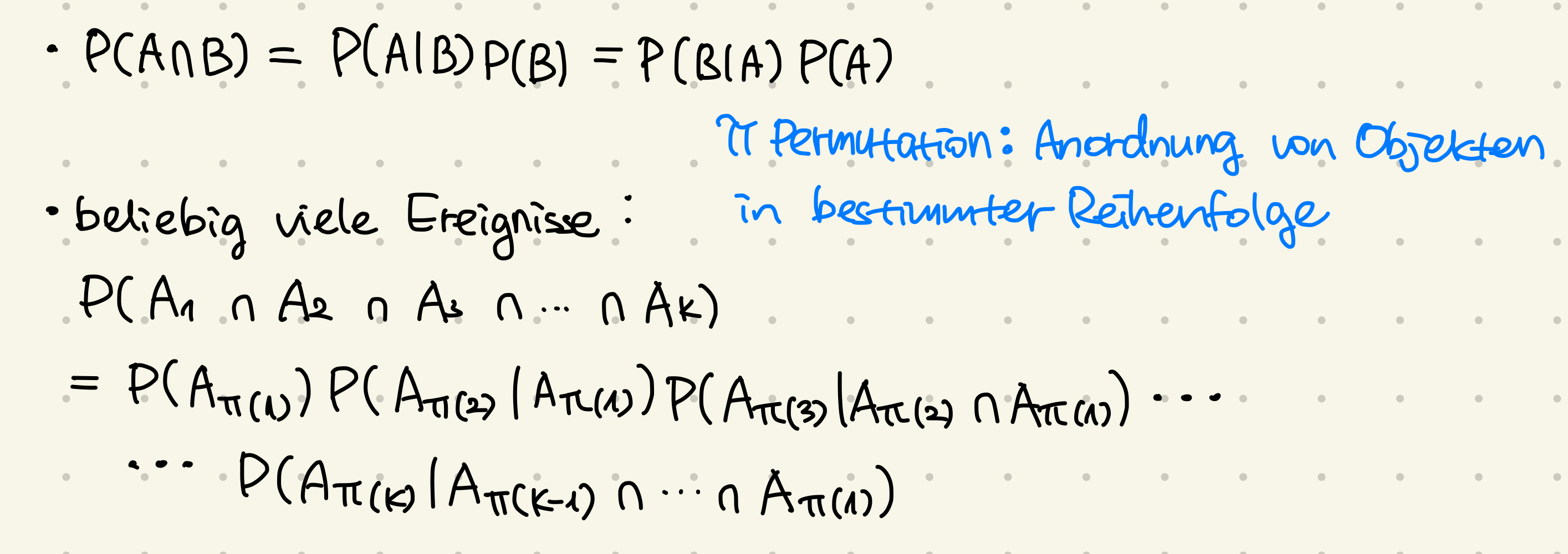
Rechenregeln für Wahrscheinlichekeitsmaß \( \mathbf{P}\) gelten für bedingte Wahrscheinlichekeiten bei gleicher Bedingung.
Beispiel
3.2 Stochastische Unabhähgigkeit von Ereignissen
Ereignisse \(\mathbf{A} \) und \(\mathbf{B} \) sind unabhängig falls:
$$ \mathbf{P}(A \cap B) = \mathbf{P}(A)\mathbf{P}(B) $$
$$ \Rightarrow \mathbf{P}(B|A) = \mathbf{P}(B) $$
Allgmein:
\( \mathbf{P} \left( \bigcap_{i \in J} A_i \right) = \prod_{i \in J} \mathbf{P}(A_i) \) mit Indenxmenge \( I\) und \( \emptyset \neq J \subseteq I \)
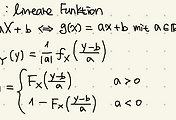


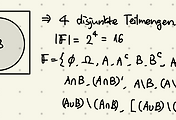
댓글